Gate Valve (G)
Gate valve in a gas network
Libraries:
Simscape /
Fluids /
Gas /
Valves & Orifices /
Flow Control Valves
Description
The Gate Valve (G) block represents an orifice with a translating gate, or sluice, as a flow control mechanism. The gate is circular and must slide perpendicular to the flow due to the constrains of the groove of its seat. The seat of the valve is annular. The flow passes through the bore, which is sized to match the gate. The overlap of the gate and the bore determines the opening area of the valve.
This image shows a gate valve with a conical seat.

The flow can be laminar or turbulent, and it can reach up to sonic speeds. The maximum velocity happens at the throat of the valve where the flow is narrowest and fastest. The flow chokes and the velocity saturates when a drop in downstream pressure can no longer increase the velocity. Choking occurs when the back-pressure ratio reaches the critical value characteristic of the valve. The block does not capture supersonic flow.
Gate valves generally open quickly. Gate valves are most sensitive to gate displacement near the closed position, where a small displacement translates into a disproportionately large change in opening area. Consequently, gate valves have too high a gain in that region to effectively throttle or modulate flow. You can use this block as a binary switch to open and close gas circuits.
Gate Mechanics
In a real valve, the gate connects by a gear mechanism to a handle. When the handle is turned from a fully closed position, the gate rises from the bore and progressively opens the valve up to a maximum. Hard stops keep the disk from breaching its minimum and maximum positions.
The block captures the motion of the disk but not the detail of its mechanics. You specify the motion as a normalized displacement at port L. The input physical signal carries the fraction of the instantaneous displacement over its value in the fully open valve.
If you want to model the action of the handle and hard stop, use Simscape mechanical blocks to capture the displacement signal. However, in many cases, it suffices to know what displacement to impart to the disk and you do not need to model the mechanics of the valve.
Gate Position
The block models the displacement of the gate but not the valve opening or closing dynamics. The signal at port L provides the normalized gate position, L. L is a normalized distance between 0 and 1, which indicates a fully closed valve and a fully open valve, respectively. If the calculation returns a number outside of this range, the block sets that number to the nearest bound.
When the Smoothing factor parameter is nonzero, the block applies numerical smoothing to the normalized gate position, L. Enabling smoothing helps maintain numerical robustness in your simulation.
For more information, see Numerical Smoothing.
Opening Area
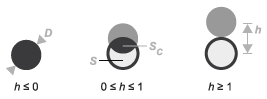
The opening area of the valve is the area of the bore adjusted for the instantaneous overlap of the gate
where:
Sopen is the instantaneous valve opening area. The block then smooths this area to remove derivative discontinuities at the limiting valve positions.
D is the common diameter of the gate and its bore, which are identical. This is the value of the Orifice diameter parameter.
SC is the area of overlap between the gate and bore, which the block computes as a function of the gate position, L:
The figure shows a front view of the valve when maximally closed, partially open, and fully open, from left to right. The figure also shows the parameters and variables in the opening area calculation.
Valve Parameterizations
The block behavior depends on the Valve parametrization parameter:
Cv flow coefficient— The flow coefficient Cv determines the block parameterization. The flow coefficient measures the ease with which a gas can flow when driven by a certain pressure differential.Kv flow coefficient— The flow coefficient Kv, where , determines the block parameterization. The flow coefficient measures the ease with which a gas can flow when driven by a certain pressure differential.Sonic conductance— The sonic conductance of the resistive element at steady state determines the block parameterization. The sonic conductance measures the ease with which a gas can flow when choked, which is a condition in which the flow velocity is at the local speed of sound. Choking occurs when the ratio between downstream and upstream pressures reaches a critical value known as the critical pressure ratio.Orifice area based on geometry— The size of the flow restriction determines the block parametrization.
The block scales the specified flow capacity by the fraction of valve opening. As the
fraction of valve opening rises from 0 to 1,
the measure of flow capacity scales from its specified minimum to its specified
maximum.
Momentum Balance
The block equations depend on the Orifice parametrization parameter.
When you set Orifice parametrization to Cv
flow coefficient parameterization, the mass flow rate, , is
where:
Cv is the value of the Maximum Cv flow coefficient parameter.
Sopen is the valve opening area.
SMax is the maximum valve area when the valve is fully open.
N6 is a constant equal to 27.3 for mass flow rate in kg/hr, pressure in bar, and density in kg/m3.
Y is the expansion factor.
pin is the inlet pressure.
pout is the outlet pressure.
ρin is the inlet density.
The expansion factor is
where:
Fγ is the ratio of the isentropic exponent to 1.4.
xT is the value of the xT pressure differential ratio factor at choked flow parameter.
The block smoothly transitions to a linearized form of the equation when the pressure ratio, , rises above the value of the Laminar flow pressure ratio parameter, Blam,
where:
When the pressure ratio, , falls below , the orifice becomes choked and the block switches to the equation
When you set Orifice parametrization to Kv flow
coefficient parameterization, the block uses these same
equations, but replaces Cv with
Kv by using the relation . For more information on the mass flow equations when the
Orifice parametrization parameter is Kv
flow coefficient parameterization or Cv flow
coefficient parameterization, [2][3].
When you set Orifice parametrization to Sonic
conductance parameterization, the mass flow rate, , is
where:
C is the value of the Maximum sonic conductance parameter.
Bcrit is the critical pressure ratio.
m is the value of the Subsonic index parameter.
Tref is the value of the ISO reference temperature parameter.
ρref is the value of the ISO reference density parameter.
Tin is the inlet temperature.
The block smoothly transitions to a linearized form of the equation when the pressure ratio, , rises above the value of the Laminar flow pressure ratio parameter Blam,
When the pressure ratio, , falls below the critical pressure ratio, Bcrit, the orifice becomes choked and the block switches to the equation
The Sonic conductance setting of the
Valve parameterization parameter is for pneumatic
applications. If you use this setting for gases other than air, you may need to
scale the sonic conductance by the square root of the specific gravity.
For more information on the mass flow equations when the Orifice
parametrization parameter is Sonic conductance
parameterization, see [1].
When you set Orifice parametrization to
Orifice area based on geometry, the mass flow
rate, , is
where:
Sopen is the valve opening area.
S is the value of the Cross-sectional area at ports A and B parameter.
Cd is the value of the Discharge coefficient parameter.
γ is the isentropic exponent.
The block smoothly transitions to a linearized form of the equation when the pressure ratio, , rises above the value of the Laminar flow pressure ratio parameter, Blam,
When the pressure ratio, , falls below , the orifice becomes choked and the block switches to the equation
For more information on the mass flow equations when the Orifice
parametrization parameter is Orifice area based on
geometry, see [4].
Mass Balance
The block assumes the volume and mass of fluid inside the valve is very small and ignores these values. As a result, no amount of fluid can accumulate in the valve. By the principle of conservation of mass, the mass flow rate into the valve through one port equals that out of the valve through the other port
where is defined as the mass flow rate into the valve through the port indicated by the A or B subscript.
Energy Balance
The resistive element of the block is an adiabatic component. No heat exchange can occur between the fluid and the wall that surrounds it. No work is done on or by the fluid as it traverses from inlet to outlet. Energy can flow only by advection, through ports A and B. By the principle of conservation of energy, the sum of the port energy flows is always equal to zero
where ϕ is the energy flow rate into the valve through ports A or B.
Assumptions and Limitations
The equation for the
Orifice area based on geometryparameterization is less accurate for gases that are far from ideal.This block does not model supersonic flow.
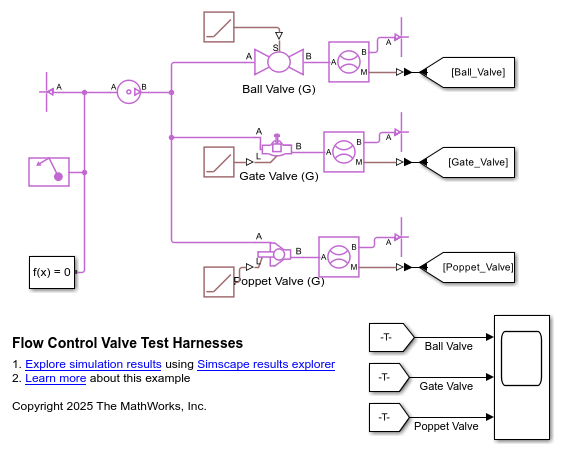
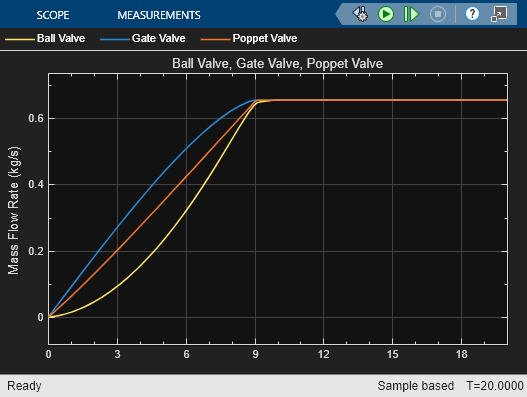
Examples
Ports
Input
Conserving
Parameters
References
[1] ISO 6358-3, "Pneumatic fluid power – Determination of flow-rate characteristics of components using compressible fluids – Part 3: Method for calculating steady-state flow rate characteristics of systems", 2014.
[2] IEC 60534-2-3, “Industrial-process control valves – Part 2-3: Flow capacity – Test procedures”, 2015.
[3] ANSI/ISA-75.01.01, “Industrial-Process Control Valves – Part 2-1: Flow capacity – Sizing equations for fluid flow underinstalled conditions”, 2012.
[4] P. Beater, Pneumatic Drives, Springer-Verlag Berlin Heidelberg, 2007.